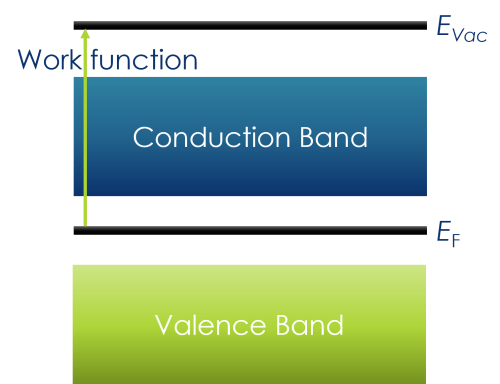
In solid state chemistry, the Fermi level is the highest electronic level when at absolute zero

Work function is the energy required to take an electron from EF to the vacuum level (E_Vac) – i.e. an electron at rest removed from the atom

In XPS, there is also an instrument work function – this will be covered later in the course

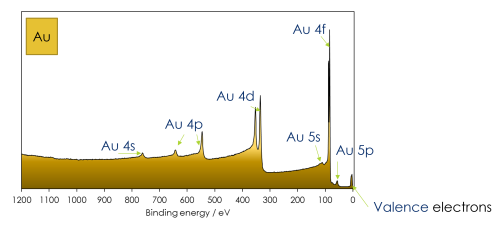
1. Binding Energy (E_B or BE) Axis: The x-axis of the spectrum is typically labeled in electronvolts (eV) and represents the binding energy of the emitted photoelectrons. Binding energy is the energy required to remove an electron from an atom or molecule within the material. The x-axis typically ranges from high binding energy (left side) to low binding energy (right side).
2. Intensity (Counts or Intensity) Axis: The y-axis represents the intensity or counts of photoelectrons detected at each energy level. It quantifies the number of emitted electrons at each binding energy.
3. Energy Regions: XPS spectra are typically divided into energy regions, each corresponding to a different type of electron transition. The main regions you’ll find in an XPS spectrum include:
   • Core-Level Peaks: These are the most prominent features in the spectrum and represent electrons from the innermost, tightly bound electron shells of the atoms. Each element has characteristic core-level binding energies, and these peaks provide information about the chemical composition of the material.
   • Valence Band: Beyond the core-level peaks, there is a region known as the valence band. It contains information about the electronic structure of the material, particularly the valence electrons involved in chemical bonding.
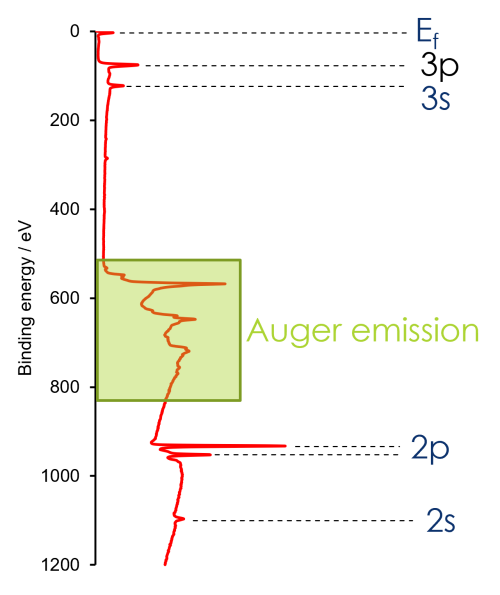
   • Auger Transitions: Auger electron peaks result from Auger transitions in which an electron falls into a core-level hole created by the ejection of a core electron. More on these later.
4. Peak Characteristics: For each core-level peak in the XPS spectrum, you can extract several key pieces of information:
   • Binding Energy (BE): The position of the peak on the binding energy axis indicates the energy required to remove an electron from that specific atomic or molecular orbital. It can be used to identify the elements present in the sample.
   • Peak Shape: The shape of the peak can provide information about the chemical state and bonding environment of the element. For example, the peak shape may be asymmetric, indicating chemical bonding or different chemical environments for the same element.
   • Peak Intensity: The height or area under the peak provides information about the concentration of the element in the sample.
5. Shifts and Splitting: XPS spectra can also show shifts in binding energies or splitting of peaks, which can be indicative of chemical interactions, chemical states, or different oxidation states of an element.
6. Background: The background signal in the XPS spectrum is usually subtracted to enhance the visibility of the peaks. It represents the inelastic scattering of electrons and other background noise.

So what are we observing?

• In XPS we are looking at the core levels closest to valence orbitals, because we use relatively soft X-rays (typically <1500 eV) to excite our photoelectrons.
• Lighter elements produce fewer peaks, since there are fewer overall orbitals within the atom.
• If we want to see deeper lying orbitals in heavier atoms, then we will need to use harder X-ray sources (HAXPES).

You can think of XPS spectra like an energy level diagram

Electrons located closer to the core bound by a higher binding energy

In reality, we are not studying a ground state but an excited state (atom following photoemission) – but energy level model a useful exercise to understanding the general spectrum

There are a number of factors affecting XPS peak intensity:

• X-ray flux (J)
• Elemental concentration (C)
• Photoionisation cross-section (σ)
• Spectrometer angular acceptance (ζ)
• Spectrometer transmission function (T)
• Inelastic mean free path (λ)

These combine to form the following expression:

I = JCσζTλ

Spectrometer transmission function and Spectrometer angular acceptance are instrumental factors that can be considered constants for the purposes of this section.

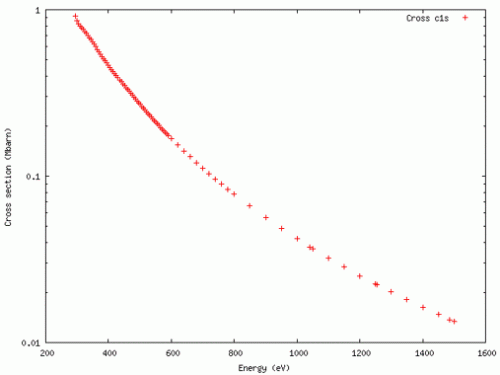
Photoionization cross sections, often denoted as σ(w), are a fundamental concept in quantum mechanics and atomic physics. They describe the probability that an incident photon will ionize an atom or molecule when it interacts with it. In other words, photoionization cross sections quantify how likely it is for a photon to remove an electron from an atom or molecule, creating a positively charged ion.

The photoionization cross section is defined as the probability per unit area that a photon with a particular energy will ionize an atom or molecule. It is usually expressed in units of square centimeters (cm²).

The photoionization cross section is a function of photon energy. This means that for different photon energies, you will have different cross sections. At certain photon energies, the cross section may have pronounced peaks corresponding to specific electron energy levels or resonances.

The photoionization cross section depends on the electron configuration of the atom or molecule being ionized. Different electron orbitals (e.g., 1s, 2s, 2p, etc.) have different ionization energies and thus different cross sections. Moreover, the angular distribution of emitted photoelectrons can vary depending on the initial electron state.

Of course, you also need enough energy to excite the electron in the first place! This is a core principle of the photoelectric effect! For example, plotting the cross sections for Ni 2p using the Elettra sincotrone photoionisation cross-section calculator – you can see that there are no cross-sections below the ionisation energy of Ni 2p electrons.

3 factors affect the width and overall distribution of electron energies around a core emission:

• X-ray energy spread  (Γ_xray)  Gaussian
• Spectrometer broadening    (Γ_spec)  Gaussian
• Intrinsic line-width  (Γ_instrinic)  Lorentzian

FWHM = (Γ_xray² + Γ_spec² + Γ_intrinsic²)^1/2

Γ_intrinsic      =     h/τ     =     4.1 x 10-15/ τ     eV

τ = core-hole lifetime (typically 10^-13 – 10^-15 s)

Intrinsic line-widths: 0.04 – 4 eV